Special Topics
Bounded forecasts
In forecasting, we often want to make sure the predictions stay within a
certain range. For example, for predicting the sales of a product, we
may require all forecasts to be positive. Thus, the forecasts may need
to be bounded.
With TimeGPT, you can create bounded forecasts by transforming your data
prior to calling the forecast function.

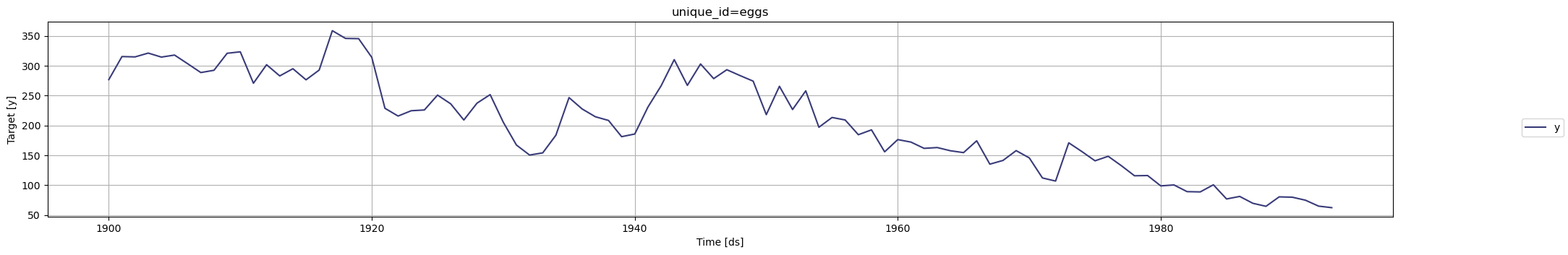
We can have a look at how the prices have evolved in the 20th century,
demonstrating that the price is trending down.

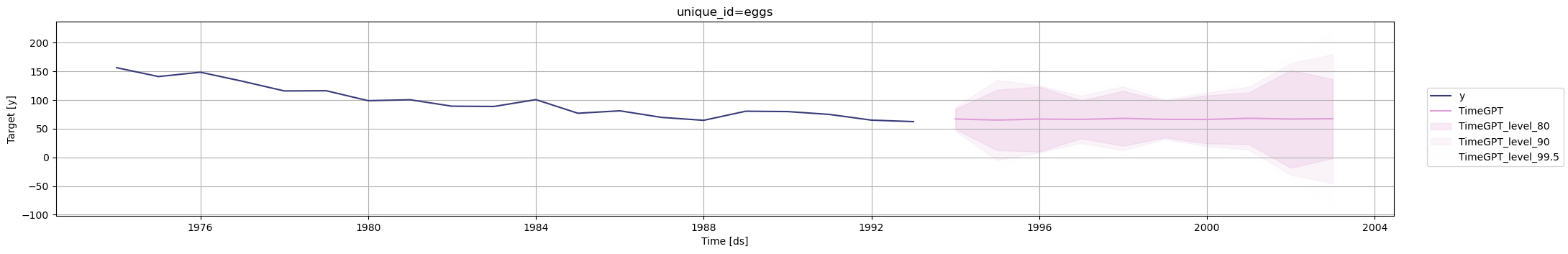
This demonstrates the value of the log-transformation to obtain bounded
forecasts with TimeGPT, which allows us to obtain better calibrated
prediction intervals.
References
1. Import packages
First, we install and import the required packages👍 Use an Azure AI endpoint To use an Azure AI endpoint, set thebase_urlargument:nixtla_client = NixtlaClient(base_url="you azure ai endpoint", api_key="your api_key")
2. Load data
We use the annual egg prices dataset from Forecasting, Principles and Practices. We expect egg prices to be strictly positive, so we want to bound our forecasts to be positive.Note You can installpyreadrwithpip:
| ds | y | unique_id | |
|---|---|---|---|
| 84 | 1984-01-01 | 100.58 | eggs |
| 85 | 1985-01-01 | 76.84 | eggs |
| 86 | 1986-01-01 | 81.10 | eggs |
| 87 | 1987-01-01 | 69.60 | eggs |
| 88 | 1988-01-01 | 64.55 | eggs |
| 89 | 1989-01-01 | 80.36 | eggs |
| 90 | 1990-01-01 | 79.79 | eggs |
| 91 | 1991-01-01 | 74.79 | eggs |
| 92 | 1992-01-01 | 64.86 | eggs |
| 93 | 1993-01-01 | 62.27 | eggs |

3. Bounded forecasts with TimeGPT
First, we transform the target data. In this case, we will log-transform the data prior to forecasting, such that we can only forecast positive prices.📘 Available models in Azure AI If you are using an Azure AI endpoint, please be sure to setAfter having created the forecasts, we need to inverse the transformation that we applied earlier. With a log-transformation, this simply means we need to exponentiate the forecasts:model="azureai":nixtla_client.forecast(..., model="azureai")For the public API, we support two models:timegpt-1andtimegpt-1-long-horizon. By default,timegpt-1is used. Please see this tutorial on how and when to usetimegpt-1-long-horizon.


| unique_id | ds | TimeGPT | TimeGPT-lo-99.5 | TimeGPT-lo-90 | TimeGPT-lo-80 | TimeGPT-hi-80 | TimeGPT-hi-90 | TimeGPT-hi-99.5 | |
|---|---|---|---|---|---|---|---|---|---|
| 0 | eggs | 1994-01-01 | 66.859756 | 43.103240 | 46.131448 | 49.319034 | 84.400479 | 87.588065 | 90.616273 |
| 1 | eggs | 1995-01-01 | 64.993477 | -20.924112 | -4.750041 | 12.275298 | 117.711656 | 134.736995 | 150.911066 |
| 2 | eggs | 1996-01-01 | 66.695808 | 6.499170 | 8.291150 | 10.177444 | 123.214173 | 125.100467 | 126.892446 |
| 3 | eggs | 1997-01-01 | 66.103325 | 17.304282 | 24.966939 | 33.032894 | 99.173756 | 107.239711 | 114.902368 |
| 4 | eggs | 1998-01-01 | 67.906517 | 4.995371 | 12.349648 | 20.090992 | 115.722042 | 123.463386 | 130.817663 |
| 5 | eggs | 1999-01-01 | 66.147575 | 29.162207 | 31.804460 | 34.585779 | 97.709372 | 100.490691 | 103.132943 |
| 6 | eggs | 2000-01-01 | 66.062637 | 14.671932 | 19.305822 | 24.183601 | 107.941673 | 112.819453 | 117.453343 |
| 7 | eggs | 2001-01-01 | 68.045769 | 3.915282 | 13.188964 | 22.950736 | 113.140802 | 122.902573 | 132.176256 |
| 8 | eggs | 2002-01-01 | 66.718903 | -42.212631 | -30.583703 | -18.342726 | 151.780531 | 164.021508 | 175.650436 |
| 9 | eggs | 2003-01-01 | 67.344078 | -86.239911 | -44.959745 | -1.506939 | 136.195095 | 179.647901 | 220.928067 |